9

In the Doctor Who episode "The End of Time Part 2",

Gallifrey is pulled out of the Time War alongside the Earth

(has it been long enough I don't need a spoiler? eh, I won't take the chance)

Gallifrey is visually several times the size of Earth, as is shown here.

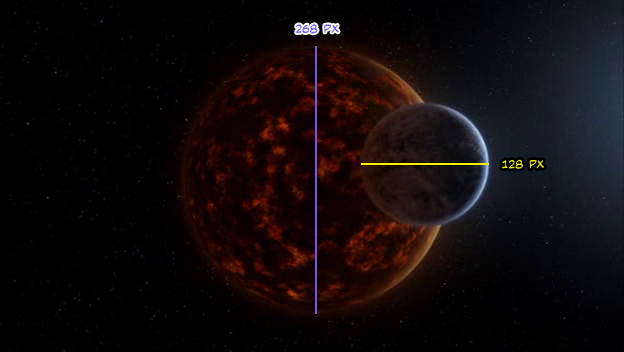Gallifrey v Earth in Pixel Lengths http://it.virtualarena.wikia.com

As you can see, Gallifrey is no laughing manner. But wouldn't Earth's orbit be pulled apart? Even for the five or ten minutes it lasts, shouldn't Gallifrey wreak havoc on Earth's orbit, as massive bodies tend to do? As the picture shows, the moon is probably gone, as Gallifrey would present a larger energy source, and thus larger attraction. Especially if it was in the way when Gallifrey made its entrance, as the picture would suggest. How does Earth escaped unscathed from this orbital confrontation?

Improve this question
11
  • 1
    5 minutes of gravity mechanics vs. millennia and you think we should slingshot off? (also spoilers don't work so well when you need photos).
    – Radhil
    Commented Jun 30, 2017 at 13:50
  • 9
    Wibbly-wobbly gravity-wavity. Don't expect DW science to always make sense.
    – Rand al'Thor ♦
    Commented Jun 30, 2017 at 13:51
  • 1
    @Radhil True on both accounts, but it's surprising what people get mad at, and if not us, definitely our moon, but lo, there it is in "Save the Moon"
    – Imperator
    Commented Jun 30, 2017 at 13:53
  • 1
    Some horrendously amateur google-math on my part says Earth might have accelerated anywhere from 8-10 m/s in some random direction (the shot isn't really clear which way Earth is being pulled). Some other amateurish googling says Earth would need maybe another 11km/s to escape solar orbit, although non-livable orbits probably need a lot less. If I could figure out how to condense mathiness into an answer, I would post this, although I am now depressed to realize I've worked this out for a show where the magic box towed Earth back to orbit not 4 episodes prior (have a +1 for that).
    – Radhil
    Commented Jun 30, 2017 at 14:29
  • 3
    Short answer really is that DW doesn't do orbital mechanics. Remember the time they dragged a bunch of planets to the same place, all close enough together to clearly see each other in the daytime sky, and there weren't any problems at all?
    – Tin Wizard
    Commented Jun 30, 2017 at 18:27

1 Answer 1

Reset to default
8

Had Gallifrey remained where it re-appeared, it would likely have shredded Earth and the Moon, and possibly sent it careening out of the solar system (or into the Sun).

That said, the same effects would have likely had similar catestrophic effects on Gallifrey. Sure, Earth is smaller, but they would both have felt tidal forces from the other, and I'm sure Gallifrey would have been at the very least battered and wrecked.

The Time Lords, however, are smart AND clever. They understand gravity in a way we don't. They have built technology that can transverse time and space in moments, and (famously) dimensional manipulation abilities that allow things to be smaller on the outside.

So I'm pretty sure the answer to "why didn't it happen" is "The Time Lords didn't want it to". There might be more specifics, like 'gravity neutralization bubble' or 'spatial impact avoidance' or other technobabble. But in the end, it didn't happen because the people who can juggle planets decided it wouldn't.

They did it for their own benefit (because putting Gallifrey back together would have been annoying, I'm sure) but they did it. And so the Earth was saved, thanks to Time Lords being surprisingly non-dickish.

Improve this answer
3
  • It's mass and distance that determine the strength of tides, not difference in size.
    – pconley
    Commented Jun 30, 2017 at 18:43
  • Granted, but that still doesn't involve the size difference between the two bodies. As far as Earthly tides are concerned, it's irrelevant whether Gallifrey is a gas giant or a point mass.
    – pconley
    Commented Jun 30, 2017 at 19:29
  • 1
    @KutuluMike isn't understanding the physics correctly. For bodies sufficiently large to be pulled to a sphere by their own gravity (like planets), the Roche limit determines where tides are strong enough to start ripping things apart. The Roche limit can be determined entirely by the densities of the two bodies and the radius of the larger body; the radius of the smaller body doesn't directly come into the equation. en.wikipedia.org/wiki/Roche_limit
    – NeutronStar
    Commented Jun 30, 2017 at 19:40

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.